Pythagorean Triple (日本語)
Less…,
A Pythagorean triple is a triple of positive integers 
, 
, and 
such that a right triangle exists with legs 
and hypotenuse 
., ピタゴラスの定理により、これは正の整数を見つけることと等価です
、
、および
満たす
 |
(1)
|
最も小さく、最もよく知られているピタゴラス三重は
です。 これらの辺の長さを持つ直角三角形は、3、4、5三角形と呼ばれることがあります。,
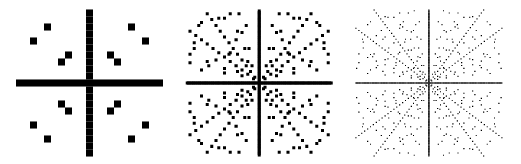
-平面内の点のプロット
がピタゴラス三重であるようなものは、連続して大きな境界について上に示されています。 これらのプロットには、
および
の負の値が含まれているため、x軸とy軸の両方について対称です。
同様に、
-平面内の点のプロットは、
がピタゴラス三重であるようなものです。,
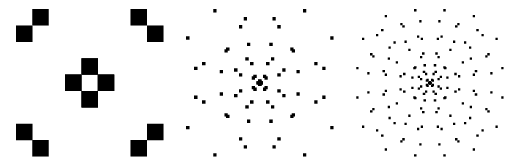
と
が比較的素数である原始的なピタゴラストリプル（”還元された”トリプルとも呼ばれる）のみを考慮するのが普通である。 プリミティブトリプルは上に示されており、元のプロットにおけるプリミティブトリプルに対応する半径方向の線は、この図には存在しないことがすぐにわかります。, プリミティブソリューションの場合、
または
のいずれかが偶数でなければならず、他の奇数（Shanks1993、p.141）で、
は常に奇数でなければなりません。,=”7a4ddb31b8″>
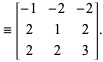
Hall (1970) and Roberts (1977) prove that 
is a primitive Pythagorean triple iff
 |
(8)
|
where 
is a finite product of the matrices 
, 
, 
.,662c5″>
Pythagoras and the Babylonians gave a formula for generating (not necessarily primitive) triples as
 |
(10)
|
for 
, which generates a set of distinct triples containing neither all primitive nor all imprimitive triples (and where in the special case 
, 
).,
The early Greeks gave
 |
(11)
|
where 
and 
are relatively prime and of opposite parity (Shanks 1993, p. 141), which generates a set of distinct triples containing precisely the primitive triples (after appropriately sorting 
and 
).
Let 
be a Fibonacci number., Then
 |
(12)
|
generates distinct Pythagorean triples (Dujella 1995), although not exhaustively for either primitive or imprimitive triples., More generally, starting with positive integers 
, 
, and constructing the Fibonacci-like sequence 
with terms 
, 
, 
, 
, 
, …, generates distinct Pythagorean triples
 |
(13)
|
(Horadam 1961), where
 |
(14)
|
where 
is a Lucas number.
For any Pythagorean triple, the product of the two nonhypotenuse legs (i.e.,、二つの小さい数）は常に12で割り切れ、すべての三辺の積は60で割り切れます。 同じ積を持つ二つの異なるトリプルがあるかどうかは知られていません。 このような二つのトリプルの存在は、ディオファントス方程式に対する非ゼロ解に対応する
 |
(15)
|
（guy1994、p.188）。,
For a Pythagorean triple (
, 
, 
),
 |
(16)
|
where 
is the partition function P (Honsberger 1985).,cdc34dc”>
(Robertson 1996).,
ピタゴラストリプルに対応する三角形の面積
あります
 |
(20)
|
フェルマーは、この形式の数が正方数になることは決してできないことを証明しました。,td>
The number of such triangles is then
 |
(22)
|
 |
(23)
|
Then
 |
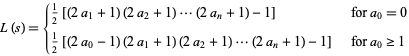(24)
|
(Beiler 1966, p., 116). なお、
iff
素数または倍の素数であることに注意してください。 の最初の数
,2,… ある0, 0, 1, 1, 1, 1, 1, 2, 2, 1, 1, 4, 1, … （OEIS A046079）。,
ウェイの数を見つけるには
数
プリミティブ直角三角形の斜辺にすることができます、その因数分解を次のように書きます
 |
(25)
|
ここで、
sは
および
sはの形式です。div id=”f2b84faf04″>
.,> as a hypotenuse is
 |
 |
 |
(29)
|
 |
 |
 |
(30)
|
(correcting the typo of Beiler 1966, p., 117、この式は非プリミティブソリューションの数のみを与えると述べています）、ここで
は二乗和関数です。, ここで、
直角三角形の脚または斜辺のいずれかであってもよい
 |
(32)
|
斜辺を持つトリプルの数をしましょう
を示す
、斜辺を持つトリプルの数
を示す
、
より小さいトリプルは、
と表されます。, Then the following table summarizes the values for powers of 10.
 |
OEIS |  ,  , … |
 |
A101929 | 1, 50, 878, 12467, … |
 |
A101930 | 2, 52, 881, 12471, … |
 |
A101931 | 1, 16, 158, 1593, ..,. |
Lehmer (1900) proved that the number of primitive solutions with hypotenuse less than 
satisfies
 |
(33)
|
(OEIS A086201).
There is a general method for obtaining triplets of Pythagorean triangles with equalareas.,b636f03a4d”>
Then the right triangle generated by each triple (
) has common area
 |
(40)
|
Right triangles whose areas consist of a single digit include 
(area of 6) and 
(area of 666666; Wells 1986, p., 89).
1643年、フェルマーはメルセンヌに対して、斜辺と足の合計が正方形であるピタゴラスの三重項を見つけることに挑戦した。,
A related problem is to determine if a specified integer 
can be the area of a right triangle with rational sides., 1、2、3、および4は有理辺直角三角形の領域ではありませんが、5は(3/2,20/3,41/6)であり、6(3,4,5)であります。, (46) has a rational solution, in which case
 |
 |
 |
(47)
|
 |
 |
 |
(48)
|
(Koblitz 1993)., 任意の
に対する解があるかどうかを判断する一般的な方法は知られていませんが、1983年にJ.Tunnellによって考案された手法により、特定の値を除外することができます(Cipra1996)。