Pythagorean Triple (한국어)
Less…,
![]()
![]()
A Pythagorean triple is a triple of positive integers ![]() ,
, ![]() , and
, and ![]() such that a right triangle exists with legs
such that a right triangle exists with legs ![]() and hypotenuse
and hypotenuse ![]() ., 여 피타고라스 정리,이에 해당을 찾는 긍정적인 정수
., 여 피타고라스 정리,이에 해당을 찾는 긍정적인 정수![]() ,
,![]() , 그리고
, 그리고![]() 만족스러운
만족스러운
|
(1)
|
작고 가장 잘 알려진 피타고라스 트리플은![]() . 이 측면 길이를 갖는 직각 삼각형을 때때로 3,4,5 삼각형이라고합니다.,
. 이 측면 길이를 갖는 직각 삼각형을 때때로 3,4,5 삼각형이라고합니다.,
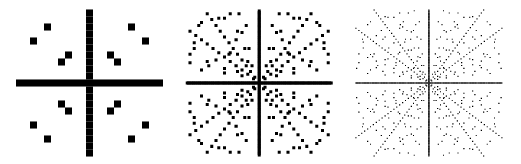
플롯의 점에서![]() -비행기는
-비행기는![]() 는 피타고라스 트리플은 다음과 같은 위한 연속적으로 큰 범위. 이러한 플롯을 포함한 부정적인 값의
는 피타고라스 트리플은 다음과 같은 위한 연속적으로 큰 범위. 이러한 플롯을 포함한 부정적인 값의![]() 및
및![]() 며,따라서 대칭에 대한 모두 x 및 y 축을 기준으로 합니다.
며,따라서 대칭에 대한 모두 x 및 y 축을 기준으로 합니다.

마찬가지로,플롯의 점에서![]() -비행기는
-비행기는![]() 는 피타고라스 트리플은 다음과 같은 위한 연속적으로 큰 범위.,
는 피타고라스 트리플은 다음과 같은 위한 연속적으로 큰 범위.,
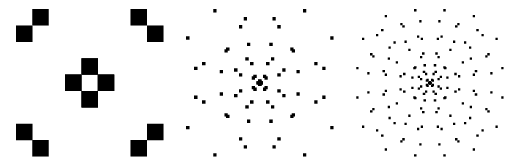
이것은 일반적인 고려할만 원시적인 피타고라스 트리플(또는”감소”세 배)에서는![]() 및
및![]() 상대적으로 주요,이후 다른 솔루션을 생성할 수 있는 이제껏에서 원시적인 것들입니다. 기본 식사를 하는 상기 그림,그리고 그것은 볼 수있는 즉시의 방사선에 해당하는 imprimitive 트리플에서 원래 그림에 존재하지 않는 이 그림입니다., 에 대한 원시적인 솔루션,하나의
상대적으로 주요,이후 다른 솔루션을 생성할 수 있는 이제껏에서 원시적인 것들입니다. 기본 식사를 하는 상기 그림,그리고 그것은 볼 수있는 즉시의 방사선에 해당하는 imprimitive 트리플에서 원래 그림에 존재하지 않는 이 그림입니다., 에 대한 원시적인 솔루션,하나의![]() 또는
또는![]() 해야 하더라도,그리고 다른 홀수(생크 1993,p. 141),
해야 하더라도,그리고 다른 홀수(생크 1993,p. 141),![]() 항상한다.,=”7a4ddb31b8″>
항상한다.,=”7a4ddb31b8″>
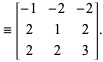
Hall (1970) and Roberts (1977) prove that ![]() is a primitive Pythagorean triple iff
is a primitive Pythagorean triple iff
|
(8)
|
where ![]() is a finite product of the matrices
is a finite product of the matrices ![]() ,
, ![]() ,
, ![]() .,662c5″>
.,662c5″>
Pythagoras and the Babylonians gave a formula for generating (not necessarily primitive) triples as
|
(10)
|
for ![]() , which generates a set of distinct triples containing neither all primitive nor all imprimitive triples (and where in the special case
, which generates a set of distinct triples containing neither all primitive nor all imprimitive triples (and where in the special case ![]() ,
, ![]() ).,
).,
The early Greeks gave
|
(11)
|
where ![]() and
and ![]() are relatively prime and of opposite parity (Shanks 1993, p. 141), which generates a set of distinct triples containing precisely the primitive triples (after appropriately sorting
are relatively prime and of opposite parity (Shanks 1993, p. 141), which generates a set of distinct triples containing precisely the primitive triples (after appropriately sorting ![]() and
and ![]() ).
).
Let ![]() be a Fibonacci number., Then
be a Fibonacci number., Then
|
(12)
|
generates distinct Pythagorean triples (Dujella 1995), although not exhaustively for either primitive or imprimitive triples., More generally, starting with positive integers ![]() ,
, ![]() , and constructing the Fibonacci-like sequence
, and constructing the Fibonacci-like sequence ![]() with terms
with terms ![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
, ![]() , …, generates distinct Pythagorean triples
, …, generates distinct Pythagorean triples
|
(13)
|
(Horadam 1961), where
|
(14)
|
where ![]() is a Lucas number.
is a Lucas number.
For any Pythagorean triple, the product of the two nonhypotenuse legs (i.e., 두 개의 작은 숫자)는 항상 12 로 나눌 수 있으며 세면 모두의 곱은 60 으로 나눌 수 있습니다. 동일한 제품을 갖는 두 개의 별개의 트리플이 있는지 알 수 없습니다. 의 존재로 이러한 두 세배에 해당하는 이 솔루션을 Diophantine equation
|
(15)
|
(남자는 1994 년,p. 188).,
For a Pythagorean triple (![]() ,
, ![]() ,
, ![]() ),
),
|
(16)
|
where ![]() is the partition function P (Honsberger 1985).,cdc34dc”>
is the partition function P (Honsberger 1985).,cdc34dc”>
(Robertson 1996).,
삼각형의 면적에 해당하는 피타고라스 트리플![]()
|
(20)
|
Fermat 증명하는 이 형태가 될 수 없 squarenumber.,td>
The number of such triangles is then
|
(22)
|
|
(23)
|
Then
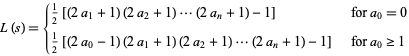 |
(24)
|
(Beiler 1966, p., 116). 참고하는![]() iff
iff![]() 은 주요 두 번니다.
은 주요 두 번니다. ![]() ,2,에 대한 처음 몇 개의 숫자… 있습니다 0, 0, 1, 1, 1, 1, 1, 2, 2, 1, 1, 4, 1, … (OEIS A046079).,
,2,에 대한 처음 몇 개의 숫자… 있습니다 0, 0, 1, 1, 1, 1, 1, 2, 2, 1, 1, 4, 1, … (OEIS A046079).,
의 번호를 찾는 방법![]() 에서는
에서는![]() 할 수 있습 빗변의 기본 바로 삼각형, 쓰고 그것의 인수 분해
할 수 있습 빗변의 기본 바로 삼각형, 쓰고 그것의 인수 분해
|
(25)
|
는![]() s 의 양식을
s 의 양식을![]() 및
및![]() s 의 양식을
s 의 양식을![]() .,> as a hypotenuse is
.,> as a hypotenuse is
|
(29)
|
|||
|
(30)
|
(correcting the typo of Beiler 1966, p., 117,이 공식은 원시적이지 않은 솔루션의 수를 제공한다고 만),여기서![]() 는 제곱 합 함수입니다., 에는
는 제곱 합 함수입니다., 에는![]() 할 수 있는 다리 또는 빗변의 삼각형에 의해 주어집
할 수 있는 다리 또는 빗변의 삼각형에 의해 주어집
|
(32)
|
자의 수를 세 겹으로 빗변![]() 로 표시
로 표시![]() 수의 세 겹으로 빗변
수의 세 겹으로 빗변![]() 로 표시
로 표시![]() , 와 숫자의 원시적 세배보다 적은
, 와 숫자의 원시적 세배보다 적은![]() 로 표시
로 표시![]() ., Then the following table summarizes the values for powers of 10.
., Then the following table summarizes the values for powers of 10.
| OEIS | |
|
| A101929 | 1, 50, 878, 12467, … | |
| A101930 | 2, 52, 881, 12471, … | |
| A101931 | 1, 16, 158, 1593, ..,. |
Lehmer (1900) proved that the number of primitive solutions with hypotenuse less than ![]() satisfies
satisfies
|
(33)
|
(OEIS A086201).

There is a general method for obtaining triplets of Pythagorean triangles with equalareas.,b636f03a4d”>
![]()
Then the right triangle generated by each triple (![]() ) has common area
) has common area
|
(40)
|
Right triangles whose areas consist of a single digit include ![]() (area of 6) and
(area of 6) and ![]() (area of 666666; Wells 1986, p., 89).
(area of 666666; Wells 1986, p., 89).
1643 년 Fermat 은 Mersenne 에게 다리의 빗변과 합이 사각형 인 피타고라스 삼중 항을 찾도록 도전했습니다.,
![]()
A related problem is to determine if a specified integer ![]() can be the area of a right triangle with rational sides., 1,2,3 및 4 는 합리적 측면 직각 삼각형의 영역이 아니지만 5 는 6(3,4,5)과 같이(3/2,20/3,41/6)입니다., (46) has a rational solution, in which case
can be the area of a right triangle with rational sides., 1,2,3 및 4 는 합리적 측면 직각 삼각형의 영역이 아니지만 5 는 6(3,4,5)과 같이(3/2,20/3,41/6)입니다., (46) has a rational solution, in which case
|
(47)
|
|||
|
(48)
|
(Koblitz 1993)., 이 없 알려진에 대한 일반적인 방법을 결정이 있을 경우 해결책에 대한 임의의![]() 지만,기술에 의해 고안 J.Tunnell1983 년에 있는 특정 값을 배제(Cipra1996).
지만,기술에 의해 고안 J.Tunnell1983 년에 있는 특정 값을 배제(Cipra1996).