Pythagorean Triple (Română)
Less…,
![]()
![]()
A Pythagorean triple is a triple of positive integers ![]() ,
, ![]() , and
, and ![]() such that a right triangle exists with legs
such that a right triangle exists with legs ![]() and hypotenuse
and hypotenuse ![]() ., Prin teorema lui Pitagora, acest lucru este echivalent cu găsirea de numere întregi pozitive
., Prin teorema lui Pitagora, acest lucru este echivalent cu găsirea de numere întregi pozitive ![]() ,
, ![]() , și
, și ![]() satisfacatoare
satisfacatoare
|
(1)
|
Cel mai mic și cel mai cunoscut teorema lui triple este ![]() . Triunghiul drept având aceste lungimi laterale este uneori numit triunghiul 3, 4, 5.,
. Triunghiul drept având aceste lungimi laterale este uneori numit triunghiul 3, 4, 5.,
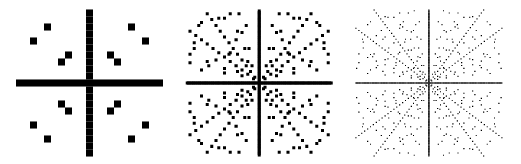
Parcele de puncte în ![]() -plan, astfel încât
-plan, astfel încât ![]() este o teorema lui triple sunt prezentate mai sus pentru succesiv mai mari limite. Aceste parcele includ valori negative ale
este o teorema lui triple sunt prezentate mai sus pentru succesiv mai mari limite. Aceste parcele includ valori negative ale ![]() și
și ![]() , și, prin urmare, sunt simetrice atât despre x – și y-axe.
, și, prin urmare, sunt simetrice atât despre x – și y-axe.

în mod Similar, parcele de puncte în ![]() -plan, astfel încât
-plan, astfel încât ![]() este o teorema lui triple sunt prezentate mai sus pentru succesiv mai mari limite.,
este o teorema lui triple sunt prezentate mai sus pentru succesiv mai mari limite.,
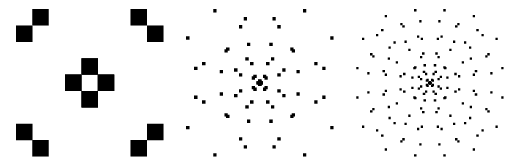
Acesta este de obicei să ia în considerare numai primitiv teorema lui triple (de asemenea, numit „redus”triple) în care ![]() și
și ![]() sunt relativ prime, de alte soluții pot fi generate în mod trivial de la cele primitive. Primitive triple sunt ilustrate mai sus, și poate fi văzut imediat că liniile radiale corespunzătoare imprimitive triple în original complot sunt absente în această figură., Pentru primitiv soluții, una dintre
sunt relativ prime, de alte soluții pot fi generate în mod trivial de la cele primitive. Primitive triple sunt ilustrate mai sus, și poate fi văzut imediat că liniile radiale corespunzătoare imprimitive triple în original complot sunt absente în această figură., Pentru primitiv soluții, una dintre ![]() sau
sau ![]() trebuie să fie chiar, iar celălalt impar (Shanks 1993, p. 141), cu
trebuie să fie chiar, iar celălalt impar (Shanks 1993, p. 141), cu ![]() întotdeauna impar.,=”7a4ddb31b8″>
întotdeauna impar.,=”7a4ddb31b8″>
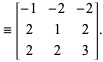
Hall (1970) and Roberts (1977) prove that ![]() is a primitive Pythagorean triple iff
is a primitive Pythagorean triple iff
|
(8)
|
where ![]() is a finite product of the matrices
is a finite product of the matrices ![]() ,
, ![]() ,
, ![]() .,662c5″>
.,662c5″>
Pythagoras and the Babylonians gave a formula for generating (not necessarily primitive) triples as
|
(10)
|
for ![]() , which generates a set of distinct triples containing neither all primitive nor all imprimitive triples (and where in the special case
, which generates a set of distinct triples containing neither all primitive nor all imprimitive triples (and where in the special case ![]() ,
, ![]() ).,
).,
The early Greeks gave
|
(11)
|
where ![]() and
and ![]() are relatively prime and of opposite parity (Shanks 1993, p. 141), which generates a set of distinct triples containing precisely the primitive triples (after appropriately sorting
are relatively prime and of opposite parity (Shanks 1993, p. 141), which generates a set of distinct triples containing precisely the primitive triples (after appropriately sorting ![]() and
and ![]() ).
).
Let ![]() be a Fibonacci number., Then
be a Fibonacci number., Then
|
(12)
|
generates distinct Pythagorean triples (Dujella 1995), although not exhaustively for either primitive or imprimitive triples., More generally, starting with positive integers ![]() ,
, ![]() , and constructing the Fibonacci-like sequence
, and constructing the Fibonacci-like sequence ![]() with terms
with terms ![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
, ![]() , …, generates distinct Pythagorean triples
, …, generates distinct Pythagorean triples
|
(13)
|
(Horadam 1961), where
|
(14)
|
where ![]() is a Lucas number.
is a Lucas number.
For any Pythagorean triple, the product of the two nonhypotenuse legs (i.e.,, cele două numere mai mici) este întotdeauna divizibil cu 12, iar produsul tuturor celor trei laturi este divizibil cu 60. Nu se cunoaște dacă există două triple distincte care au același produs. Existența a două astfel de triple corespunde la o soluție diferită de zero la Diophantine ecuație
|
(15)
|
(Tip 1994, p. 188).,
For a Pythagorean triple (![]() ,
, ![]() ,
, ![]() ),
),
|
(16)
|
where ![]() is the partition function P (Honsberger 1985).,cdc34dc”>
is the partition function P (Honsberger 1985).,cdc34dc”>
(Robertson 1996).,
aria unui triunghi care corespunde lui Pitagora triplu ![]()
|
(20)
|
Fermat s-a dovedit că un număr de această formă nu poate fi niciodată un squarenumber.,td>
The number of such triangles is then
|
(22)
|
|
(23)
|
Then
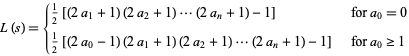 |
(24)
|
(Beiler 1966, p., 116). Rețineți că ![]() iff
iff ![]() este prim sau de două ori pe prime. Primele câteva numere pentru
este prim sau de două ori pe prime. Primele câteva numere pentru ![]() , 2,… sunt 0, 0, 1, 1, 1, 1, 1, 2, 2, 1, 1, 4, 1, … (OEIS A046079).,
, 2,… sunt 0, 0, 1, 1, 1, 1, 1, 2, 2, 1, 1, 4, 1, … (OEIS A046079).,
Pentru a găsi numărul de moduri ![]() în care un număr
în care un număr ![]() poate fi ipotenuza unui primitiv triunghi dreptunghic, scrie factorizarea ca
poate fi ipotenuza unui primitiv triunghi dreptunghic, scrie factorizarea ca
|
(25)
|
unde ![]() – uri sunt de forma
– uri sunt de forma ![]() și
și ![]() – uri sunt de forma
– uri sunt de forma ![]() .,> as a hypotenuse is
.,> as a hypotenuse is
|
(29)
|
|||
|
(30)
|
(correcting the typo of Beiler 1966, p., 117, care afirmă că această formulă dă numărul de soluții non-primitive numai), unde ![]() este suma funcției pătrate., în care
este suma funcției pătrate., în care ![]() poate fi un picior sau ipotenuza unui triunghi dreptunghic este dat de
poate fi un picior sau ipotenuza unui triunghi dreptunghic este dat de
|
(32)
|
Lasa numarul de triplete cu ipotenuza ![]() fi notate
fi notate ![]() , numărul de triplete cu ipotenuza
, numărul de triplete cu ipotenuza ![]() fi notate
fi notate ![]() , și numărul de primitiv triple mai puțin decât
, și numărul de primitiv triple mai puțin decât ![]() fi notate
fi notate ![]() ., Then the following table summarizes the values for powers of 10.
., Then the following table summarizes the values for powers of 10.
| OEIS | |
|
| A101929 | 1, 50, 878, 12467, … | |
| A101930 | 2, 52, 881, 12471, … | |
| A101931 | 1, 16, 158, 1593, ..,. |
Lehmer (1900) proved that the number of primitive solutions with hypotenuse less than ![]() satisfies
satisfies
|
(33)
|
(OEIS A086201).

There is a general method for obtaining triplets of Pythagorean triangles with equalareas.,b636f03a4d”>![]()
Then the right triangle generated by each triple (![]() ) has common area
) has common area
|
(40)
|
Right triangles whose areas consist of a single digit include ![]() (area of 6) and
(area of 6) and ![]() (area of 666666; Wells 1986, p., 89).în 1643, Fermat l-a provocat pe Mersenne să găsească un triplet pitagorean a cărui ipotenuză și suma picioarelor erau pătrate.,
(area of 666666; Wells 1986, p., 89).în 1643, Fermat l-a provocat pe Mersenne să găsească un triplet pitagorean a cărui ipotenuză și suma picioarelor erau pătrate.,![]()
A related problem is to determine if a specified integer ![]() can be the area of a right triangle with rational sides., 1, 2, 3 și 4 nu sunt zonele oricărui triunghi drept rațional, dar 5 este (3/2, 20/3, 41/6), așa cum este 6 (3, 4, 5)., (46) has a rational solution, in which case
can be the area of a right triangle with rational sides., 1, 2, 3 și 4 nu sunt zonele oricărui triunghi drept rațional, dar 5 este (3/2, 20/3, 41/6), așa cum este 6 (3, 4, 5)., (46) has a rational solution, in which case
|
(47)
|
|||
|
(48)
|
(Koblitz 1993)., Nu se cunoaște nici o metodă generală pentru a determina dacă există o soluție pentru arbitrare ![]() , dar o tehnică elaborată de J. Tunnell în 1983 permite ca anumite valori să fie exclus (Cipra 1996).
, dar o tehnică elaborată de J. Tunnell în 1983 permite ca anumite valori să fie exclus (Cipra 1996).